Exercises 18 (Polar coordinates)

  1. Express in polar form:

    1. \((5, 2)\)
    2. \((3, 7)\)
    3. \((-5, 2)\)
    4. \((-8, -9)\)
    5. \((17, -12)\)

  2. Express in rectangular form:

    1. \(5 \angle 65^\circ\)
    2. \(3.8 \angle 124^\circ\)
    3. \(7.2 \angle -56^\circ\)
    4. \(15 \angle -138^\circ\)

  3. Six holes are marked out in polar coordinates as:

Hole 1 \(65 \angle 65^\circ\)
Hole 2 \(55 \angle 95^\circ\)
Hole 3 \(45 \angle -20^\circ\)
Hole 4 \(80 \angle 55^\circ\)
Hole 5 \(160 \angle -170^\circ\)
Hole 6 \(95 \angle -80^\circ\)

Sketch the system and determine the position of Hole 1 relative to Hole 6 in rectangular coordinates. The coordinates for each hole are given relative to the previous hole.

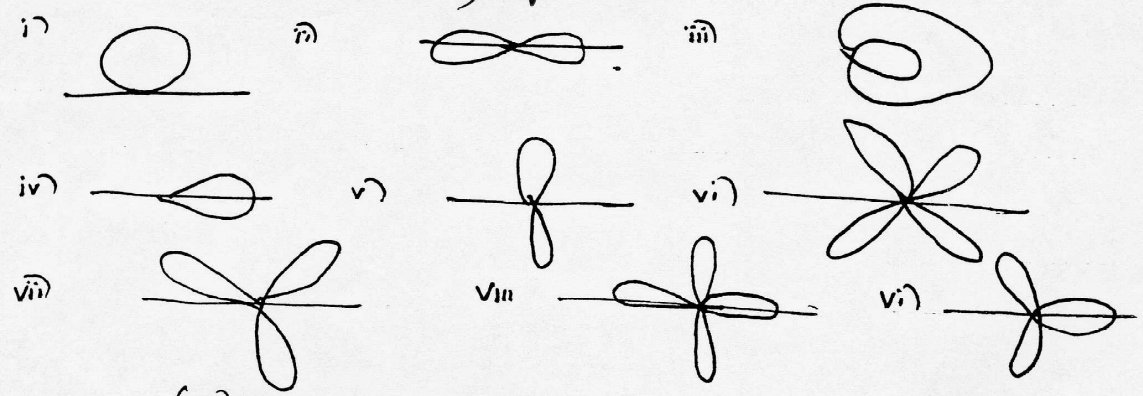
  1. For values of \(\theta\) in the range \(0\) to \(2\pi\), plot at intervals of \(\pi/6\) radians the functions:

    1. \(r = 2 \sin \theta\)
    2. \(r = 2 \cos^2\theta\)
    3. \(r = a(1 + 2 \cos \theta)\)
    4. \(r = a \cos \theta\)
    5. \(r = a \sin^2\theta\)
    6. \(r = a \sin 2\theta\)
    7. \(r = a \sin 3\theta\)
    8. \(r = a \cos 2\theta\)
    9. \(r = a \cos 3\theta\)

Assume \(a\) is a constant.

Solutions: 1. (i) \(5.38\angle21.8^\circ\); (ii) \(7.61\angle66.8^\circ\); (iii) \(5.38\angle158.2^\circ\); (iv) \(12.04\angle-131.6^\circ\); (v) \(20.8\angle -35.23^\circ\); 2. (i) \((2.1,4.3)\); (ii) \((-2.1,3.15)\); (iii) \((4.03,-5.97)\); (iv) \((-11.1,-10.0)\); 3. \((57.7,16.4)\); 4.